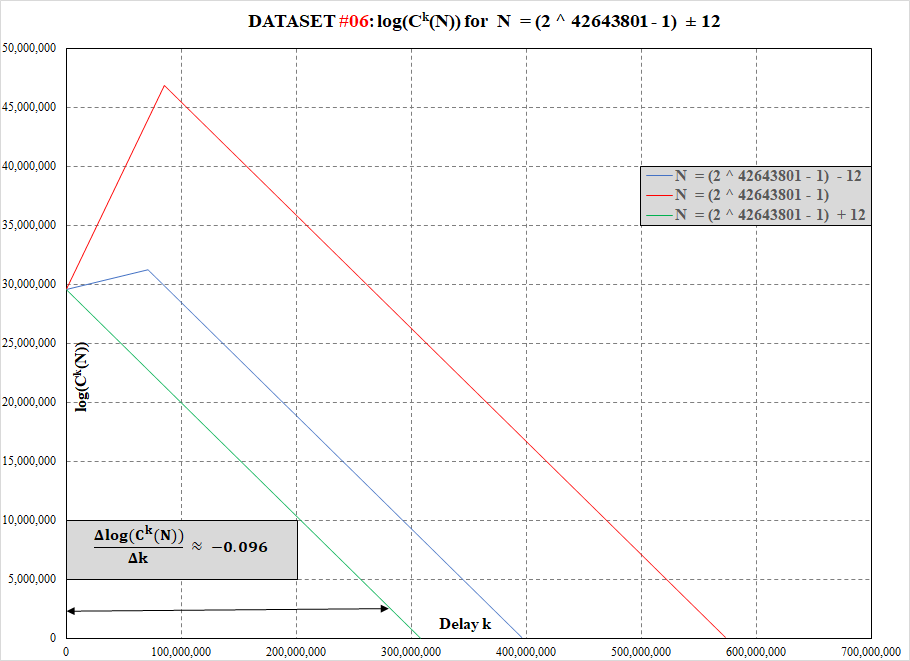
We publish further data on the Collatz function for 242643801 – 1 (#6 known megaprime) and two of its odd neighbors located at ±12.
Once the maximum is reached, the speed of decline for the log of the Collatz function seems to be the same for all tested numbers and is equal to approximately -0.096.